Grade X Maths Term-2 Important Questions
CBSE 10th Term-2 2022 : Mathematics Important 2 Marks Questions with Solution
Check important very short answer type questions have been prepared by the examination experts. Students can easily read all questions in revise them to score maximum marks in their Maths exam. Students are requested to go through it properly to boost your term-2 marks
Chapter - Quadratic Equations
Que 1. Find the value(s) of p for which the quadratic equation 2x2 + 3 x − p = 0 has real roots.
Ans 1. The given quadratic equation is,
2x2 + 3 x − p = 0
Here,
a = 2, b = 3, c = −p
If the equation is supposed to have real roots, then D≥0. Therefore,
b2 − 4ac ≥ 0
9 − 4 × 2 × (−p) ≥ 0
9 + 8p ≥ 0
p ≤ 9/8
Thus, the values of p for which the equation has real roots are (−∞,9/8].
Que 2. One year ago, a man was 8 times as old as his son. Now his age is equal to the square of his son's age. Then find their present ages
Ans 2. Let x be the age of the son one year ago, then the age of the man was 8x.
The present age of the son is (x + 1) and that of the man is (8x + 1). Then,
8x + 1 = (x + 1)2
8x + 1 = x2 + 1 + 2x
x2 = 6x
x = 0,6
As the age cannot be 0, so the value of x is 6.
So, present age of son = (x + 1) = 7 years and present age of man = (8x + 1) = 49 years
Que 3. Find the sum of the roots of the quadratic equation 3x2 - 9x + 5 = 0?
Ans 3.
Given,
The equation as 3x2 - 9x + 5 = 0
To find,
Sum of the roots in the quadratic equation
Solution,
Let's find the roots for the given equation first.
3x2 - 9x + 5 = 0
Quadratic roots formula is,
Now, lets add the roots, we get 18/6 = 3
Hence, sum of the roots for given equation is 3.
Que 4. If 1/2 is a root of the equation x2 + kx - 5/4 = 0 then find the value of k?
Ans 4. Given that,
1/2 is a root of the equation, x2 + kx - 5/4 = 0
To find,
The value of k.
Solution,
If 1/2 is the root of given equation, then it will satisfy it. It means put x = 1/2 in the given equation.
So,
Hence, the value of k is 2.
Que 5. .A natural number, when increased by 12, equals 160 times its reciprocal. Find the number?
Ans 5. Let the number be x. Then,
x + 12 = 160 × 1/x
x2 + 12x − 160 = 0
(x + 20)(x − 8) = 0
x = −20,8
Therefore, the required number is 8.
Chapter - Arithmetic Progressions
Que 1. How many two digits numbers are divisible by 3?
Ans 1. First two digit number divisible by 3 = 12
Last two digit number divisible by 3 = 99
An arithmetic progression (A.P) is a progression in which the difference between two consecutive terms is constant.
A.P = 12,15,18,…,99
here
First term (a) = 12
Common difference (d) = 3
Let us consider there are n numbers then
an = 99
a + (n – 1)d = 99
12 + (n – 1)3 = 99
12 + 3n – 3 = 99
n = 29+1
n = 30
∴ Two digit numbers divisible by 3 = 30.
Que 2. In an AP, if the common difference (d) = -4 and the seventh term (a7) is 4, then find the the first term.
Ans 2. we know,an = a + (n−1)d
so, a7 = a + (7−1)d
or, 4 = a + 6 (−4)
or, 24 + 4 = a
∴ a = 28
Que 3. Which term of AP 8,14,20,26...will be 72 more than its 41st term?
Ans 3. Step-by-step explanation:
Given :
8, 14, 20, 26, ..
first term , a = 8 , common difference , d = 14 - 8 = 6
nth term = 72 + a41
a + (n -1)d = 72 + a41
[nth term = a + (n -1)d]
8 + (n - 1) 6 = 72 + a + (41 - 1) d
8 + 6n - 6 = 72 + 8 + 40 × 6
2 + 6n = 72 + 8 + 240
2 + 6n = 80 + 240
2 + 6n = 320
6n = 320 - 2
6n = 318
n = 318/6
n = 53
Hence, 53rd term of the A.P 8, 14, 20, 26, ... will be 72 more than its 41st term.
Que 4. Write the nth term of A.P. 1/m, (1+m)/m, (1+2m)/m, ……..?
Ans 4. We know that,
Que 5. Find the middle term of 6, 13, 20... 216?
Ans 5. The given AP is 6,13,20,........,216.
First term, a = 6
Common difference d = 13-6 = 7
Suppose these are n terms in the given AP. Then,
Hence, the middle term of the given AP is 111.
Que 6. The 8th term of AP is zero . Prove that 38th term is triple of 18th term.
Ans 6. T8 = a + 7d = 0
⇒ a = −7d
T38 = a + 37d
= −7d + 37d
= 30d
T18 = a + 17d
= −7d + 17d
= 10d.
Clearly T38=3(T18).
Que 7. Find the sum of all two digit positive odd numbers?
Ans 7. All two-digit odd positive numbers are 11, 13, 15, 17, ...., 99. which are in AP with a = 11, d = 2, l = 99
Let the number of terms be n.
an = 99
a + (n − 1)d = 99
11 + (n − 1) × 2 = 99
n = 45
Sum of n terms is given by
Sn = n/2(a + l)
Sn = 45/2(11 + 99) = 2475
Therefore, the sum of all two-digit odd positive numbers is 2475.
Que 8. Three numbers are in AP and their sum is 21, find the middle number ?
Ans 8. Let the numbers be,
a − d, a, a + d
Given,
a − d + a + a + d = 21
3a = 21
∴ a = 7
Hence the middle number.
Que 9. If 7 times the 7th term of an AP is equal to 11 times its 11th term. Then find its 18th term.
Ans 9. Let a and d be first term and common difference respectively
According to the question,
7 times the 7th term of an AP is equal to 11 times its 11th term
⇒ 7a7 = 11a11
As we know, nth term of an AP is
an = a + (n - 1)d
where a = first term
an is nth term
d is the common difference
we have,
7(a + 6d) = 11(a + 10d)
⇒ 7a + 42d = 11a + 110d
⇒ 4a + 68d = 0
⇒ a + 17d = 0
⇒ a18 = 0
18th term of AP is 0
Que 10. The consecutive terms of an AP are 2, x, 26, find the value of x?
Ans 10. x = 14
Step-by-step explanation:
2, x, 26 are in AP.
Hence,
x - 2 = 26 - x
2x = 28
x = 14
Que 11. For what value of p is 2p+1, 13, 5p-3, are 3 consecutive terms of an AP?
Ans 11. Given 2p+1, 13 , 5p-3 are in AP.
To find: value of P
If a,b,c are in AP then we know that
2b = ( a+c)
Here a = 2p + 1
b = 13
c = 5p - 3
2 (13) = (2p+1+5p-3)
26 = 7p-2
26 + 2 = 7p
28 = 7p
p = 28/7 = 4
Therefore the value of P is 4.
Que 12. Which term of the AP: 3, 8, 13, 18... is 78?
Ans 12. The formula for nth term of an AP is aₙ = a + (n - 1) d
The given arithmetic progression is 3, 8, 13, 18, ...
First term: a = 3
Second term: a + d = 8
Common difference: d = 8 - 3 = 5
an = a + (n - 1) d
an = 78, n = ?
3 + (n - 1) 5 = 78
5(n - 1) = 78 - 3
n - 1 = 15
n = 16
78 is the 16th term of the given AP.
Que 13. Write the 5th term from the end of the AP 3,5,7,9 .. 201?
Ans 13. Step-by-step explanation:
Given :
A.P. 3, 5, 7, 9, ....,201.
Let 'l' be the last term of an A.P
Here, l = 201, a = 3, a2 = 5
d = a2 – a
d = 5 - 3 = 2
nth term from the end = l - (n - 1)d
5th term from the end = l - (n - 1)d
5th term from the end = 201 - (5 - 1) × 2
5th term from the end = 201 - 4 × 2
5th term from the end = 201 - 8
5th term from the end = 193
Hence, the 5th term from the end of the A.P is 193.
Que 14. From the given AP: 8, 10, 12... Find the sum of its last 10 terms if it has 60 terms?
Ans 14. A.P is 8,10,12......................
Then first term is 8 and common difference is 2.
an = a + (n−1)d.
Then a60 = 8 + (60−1)2 = 8 + 59 × 2 = 126
Last 10 term =a51, a52..................a60
Then a51 = 8 + (51 − 1)2 = 108
So total of last 10 terms = 10/2 [108 × 2 + (10 − 1)2] = 216 + 81 = 234 × 5 = 1170
Que 15. Find the number of terms of an AP 5, 9, 13 ...185.
Ans 15. Step-by-step explanation:
Given:
l = 185
a = 5
d = 4
To find:
n = ?
Solution:
l = a + (n - 1)d
185 = 5 + (n - 1)4
185 = 5 + 4n -4
185 = 1 + 4n
185 - 1 = 4n
184 = 4n
184/4 = n
46 = n
Chapter - Circles
Que 1. Prove that the line segments joining the points of contact of two parallel tangents is a diameter of the circle.
Ans 1. Consider the circle with center at O
PQ & RS are two parallel tangents to it touching at A and B respectively.
Join OA and OB
Now OA perpendicular to OQ (∴ radius is perpendicular to tangent)
And OB perpendicular to RS
∴ OA ∥ OB
But OA and OB pass through O
∴ AB is straight line through center
∴ AB is a diameter
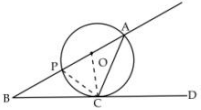
Que 2. O is the centre of the circle and BCD is a tangent to it at C. Prove that ∠BAC + ∠ACD = 90 0.
Ans 2. Given: In the above figure, O is the centre of the circle and BCD is tangent to it at C.
To prove: ∠BAC + ∠ACD = 90°
Proof: In ΔOAC
OA = OC [radii of same circle]
⇒ ∠OCA = ∠OAC [angles opposite to equal sides are equal]
⇒ ∠OCA = ∠BAC [1]
Also,
OC ⊥ BD [Tangent at any point on a circle is perpendicular to the radius through point of contact]
⇒ ∠OCD = 90°
⇒ ∠OCA + ∠ACD = 90°
⇒ ∠BAC + ∠ACD = 90° [From 1]
Hence Proved
Que 3. In the figure quadrilateral, ABCD is drawn to circumscribe a circle. Prove that AD+BC = AB + CD.
Ans 3. Given:- Let ABCD be the quadrilateral circumscribing the circle with centre O. The quadrilateral touches the circle at point P,Q,R and S.
To prove:- AB + CD + AD + BC
Proof:-
As we know that, length of tangents drawn from the external point are equal.
Therefore,
AP = AS.....(1)
BP = BQ.....(2)
CR = CQ.....(3)
DR = DS.....(4)
Adding equation (1),(2),(3) and (4), we get
AP + BP + CR + DR = AS = BQ + CQ + DS
(AP + BP) + (CR + DR) = (AS + DS) + (BQ + CQ)
⇒ AB + CD = AD + BC
Hence proved.
Que 4. Prove that the tangents drawn at the end-points of the diameter of a circle are parallel.
Ans 4. To prove: PQ ∣∣ RS
Given: A circle with centre O and diameter AB. Let PQ be the tangent at point A & Rs be the point B.
Proof: Since PQ is a tangent at point A.
OA ⊥ PQ(Tangent at any point of circle is perpendicular to the radius through point of contact).
∠OQP = 90o …………(1)
OB ⊥ RS
∠OBS = 90o ……………(2)
From (1) & (2)
∠OAP = ∠OBS
i.e., ∠BAP = ∠ABS
for lines PQ & RS and transversal AB
∠BAP = ∠ABS i.e., both alternate angles are equal.
So, lines are parallel.
therefore PQ || RS.
Que 5. Two concentric circles have centre O, OP = 4cm, OB = 5cm. AB is a chord of the outer circle and tangent to the inner circle at P. Find the length of AB.
Ans 5.
OP = 4 cm, OB = 5 cm
We know that the radius is perpendicular to the tangent at the point of contact.
∠OPB = 90o
In right triangle OPB,
OB2 = OP2 + PB2
(5)2 = (4)2 + PB2
PB2 = 25 - 16 = 9
PB = 3 cm
We know that perpendicular from the centre to the chord bisect the chord.

Que 6. Two tangents PA and PB are drawn to a circle with centre O such that ∠APB=120o. Prove that OP = 2AP
Ans 6. O is the center of the given circle
∠OAP = ∠OBP = 90o
(Radius is perpendicular to the tangent at the point of contact)
OA = OB
(radius of the circle)
∴ △OAP is congruent to △OBP
So that,
∠OPA = ∠OPB = 120/2 = 60o
In △OAP
cos∠OPA = cos60o = AP/OP {cos60o = 1/2}
1/2 = AP/OP
OP = 2AP Hence proved
Que 7. In the isosceles triangle ABC in fig. AB = AC, show that BF = FC.
Ans 7. ABC is an isosceles triangle (given) AB = AC (given) BE and CF are two medians (given) To prove: BE = CF In △CFB and △BEC CE = BF (Since, AC = AB = AC/2 = AB/2 = CE = BF) BC = BC (Common) ∠ECB = ∠FBC (Angle opposite to equal sides are equal) By SAS theorem: △CFB ≅ △BEC So, BE = CF (By c.p.c.t)
Que 8. In the fig. a circle is inscribed in a ∆ABC with sides AB = 12cm, BC = 8 cmand AC=10cm. Find the lengths of AD, BE and CF.
Ans 8. We know that AD = AF
BD = BE
CE = CF
Let AD = AF = x
BD = BE = y
CE = CF = z
Then x + y = 12
y + z = 8
x + z = 10
On Solving above equation we get x = 7,y = 5,z = 3
So AD = 7 , BE = 5 , CF = 3
Que 9. In fig. circle is inscribed in a quadrilateral ABCD in which ∠B = 90o . If AD = 23cm, AB = 29cm, and DS = 5cm, find the radius ‘r’ of the circle.
Ans 9. In the figure. AB, BC, CD and DA are the tangents drawn to the circle at Q, P, S and R respectively.
∴ DS = DR (tangents drawn from a external point D to the circle).
but DS = 5 cm (given)
∴ DR = 5 cm
In the fig. AD = 23 cm, (given)
∴ AR = AD - DR = 23 - 5 = 18 cm
but AR = AQ
(tangents drawn from an external point A to the circle)
∴ AQ = 18 cm
If AQ = 18 cm then (given AB = 29 cm)
BQ = AB - AQ = 29 - 18 = 11 cm
In quadrilateral BQOP,
BQ = BP (tangents drawn from an external point B)
OQ = OP (radii of the same circle)
∠QBP=∠QOP=90o (given)
∠OQB=∠OPB=90o (angle between the radius and tangent at the point of contact.)
∴ BQOP is a square.
∴ radius of the circle, OQ = 11 cm
Que 10. In fig. two circles touch each other externally at C. Prove that the common tangent at C bisects the other two tangents.
Ans 10. We know that lengths drawn from an external point to a
circle are equal
RP = RC and RC = RQ
RP = RQ
R is the mid-point of PQ.
RP - RO
R is the mid-point of PQ,
Chapter - Constructions
Que 1. Draw a line segment of length 8.4 cm and divide it in the ratio 2:5? Measure their lengths?
Ans 1. Step 1 : Draw a line segment AB = 8.4 cm
Step 2 : Draw an acute angle BAC
Step 3 : Using suitable compass width take 7 points on AC, one by one at equal distance.
Step 4 : Join 7th point with B
Step 5 : Draw a line parallel to line in previous step, passing through 2nd point and intersecting AB at M.
M divides AB in 2 : 5 ratio
AM.= 2.4 cm
MB = 6 cm
2.4 : 6
2:5
Que 2. Draw a circle of radius 4cm. From a point 8cm away from its centre, construct pair of tangents to the circle.
Ans 2. Step-by-step explanation:
1. draw a circle with centre 'O' of radius 4cm.
2. take a point 'P' 8 cm away from its centre.
3. join OP. draw perpendicular bisector of OP.
4. name the point of intersection as M.
5. taking MP as radius and M as centre draw another circle.
6. name the point A and B where the smaller circle intersects the larger circle respectively.
7. PA and PB are the required tangents.
Chapter - Some Applications Of Trigonometry
Que 1. An airplane at an altitude of 200m observes the angles of depression of opposite points on the two banks of a river are to be 45o and 60o. Find the width of the river. (Take √3=1.73)
Ans 1.
In the figure, C denotes the position of the aeroplane. Points A and B denotes the position of the two points on two banks of the river.
We have to find AB, i.e., a + b
In △ ACD,
Que 2. A tree 12m high is broken by the wind in such a way that its top touches the ground and makes an angle 60o with the ground. Find the height from the bottom of the tree is broken by the wind. (Take √3=1.73)
Ans 2.
Que 3. At some time of the day the length of the shadow of a tower is equal to its height. Find the sun’s altitude at that time.
Ans 3. Let the length of shadow = x
⇒ Height = x
Angle = A
so, tanA = x/x = 1
tanA=tan450
so, A=450
Hence, the answer is 450.
Que 4. A ladder 15m long makes an angle of 600 with the wall. Find the height of the point where the ladder touches the wall.
Ans 4.
From figure,
cos60o = x/15
⇒ 1/2 = x/15
⇒ x = 15/2m
The height of the point where the ladder touches the wall is 7.5 m
Que 5. A vertical pole 20m long casts a shadow 20√3m long. Find the sun’s altitude. At the same time a tower casts a shadow 90m long. Determine the height of the tower.
Ans 5. In ΔABC & ΔADE
∠BCA = ∠DEA = 90o [Each are at 90o to the ground]
∠BAC = ∠DAE [common angle]
∠CBA = ∠EDA [∵ the sum will cast light a equal angles]
Hence, by AAA, ΔABC∼ΔADE
⇒ CABC = EADE
⇒ 1020 = 50DE
⇒ DE = 100
∴ The height of the tower is 100m.
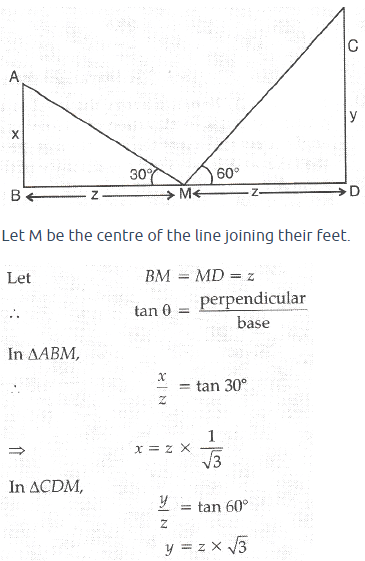
Que 6. The tops of two towers of heights x and y standing on level ground, making angles 30o and 60o respectively at the Centre of the line joining their feet. Find x:y.
Ans 6.
Que 7. From a balloon vertically above a straight road, the angles of depression of two cars at an instant are found to be 45o and 60o . If the cars are 100m apart, find the height of the balloon
Ans 7. Let the height of the balloon at P be h meters (see Fig. 8.4). Let A and B be the two cars. Thus AB = 100 m. From ΔPAQ, AQ = PQ = h
Que 8. The angle of elevation of the top of the first storey of a building is 30o at a point on the ground distance 15m from its foot. How high its second storey will be if the angle of elevation of the top of the second storey at the same point is 45o .
Ans 8.
Let DAB be the 2 storeys in the building with 2nd storey at D and 1st storey
at A. B is the foot of the building.
Let C be the point on the ground 15 m away from the buildings foot.
Now, given that angle of elevation of top of first storey is 30°,
tan ∠ACB = AB/BC
=> tan 30° = AB/15
=> AB = 15/√3
=> AB = 5√3.
Hence the first storey is 5√3 m high.
Angle of elevation of the top of second storey is given as 45°
i.e., ∠DCB = 45°
=> tan 45° = DB/BC
=>1 = DB/15
=>DB = 5m.
Hence the second storey is 15 m high.
Que 9. From a bridge, 25m high, the angle of depression of a boat is 45o . Find the horizontal distance of the boat from the bridge.
Ans 9.
Let the angle B be 45 degree (Angle of depression)
when B is 45 degree then angle A will also be 45 degrees.
Let a be the height of the bridge=25 m(given)
Let b be the distance from the boat to the bridge.
therefore tan teta=opposite side/adjacent side .(teta=45degrees) tan 45 degree= a/b
(tan 45 degree=1) --(standard unit)
then tan 45 degrees = a/b
= 1 =25/b
therefore on solving , b=25m which is the distance from boat to the bridge.
Que 10. A 1.8m tall girl stands at a distance of 4.6m from a lamp post and casts a shadow of 5.4m on the ground. Find the height of the lamp post.
Ans 10.
Using trigonometric ratios,
Let AB be the height of lamp post.
Now, in right △CDE,
⇒ tanθ= ED/DC
= 1.8 / 5.4
= 1/3 ⟶(1)
In , △ACB,
⇒ tanθ= AB/BC
= AB/ 4.6+5.4
=AB/10 ⟶ (2)
From (1) & (2) we get,
⇒ 1/3 = AB/10
⇒AB= 10/3
∴ Height of the lamp post =10/3 m
Chapter - Surface Areas and Volumes
Que 1. A sphere of diameter 18 cm is dropped into a cylindrical vessel of diameter 36cm, partly filled with water. If the sphere is completely submerged, then calculate therise of water level (in cm).
Ans 1.
Que 2. Find the number of solid spheres, each of diameter 6 cm that can be made by melting a solid metal cylinder of height 45 cm and diameter 4 cm.
Ans 2. Let the number of spheres = n
Radius of sphere = 3 cm, Radius of cylinder = 2 cm
Volume of spheres = Volume of cylinder
Number of solid spheres = 5
Que 3. Volume and surface area of a solid hemisphere are numerically equal. What is the diameter of hemisphere?
Ans 3.
Que 4. If the total surface area of a solid hemisphere is 462 cm2 , find its volume. (π= 3.14)
Ans 4. Given, TSA of hemisphere = 462 cm2
Que 5. Two cubes, each of side 4 cm are joined end to end. Find the surface area of the resulting cuboid.
Ans 5. two cubes are joined end to end means
breadth = 4cm no change
height = 4cm. no change
but
length = 4cm + 4cm + 8cm
put all the values in the formulae
AREA OF CUBOID = 2(L × B + B × H + H × L)
AREA OF CUBOID = 2(8 × 4 + 4 × 4 + 4 × 8)
AREA OF CUBOID = 2(80)
AREA OF CUBOID = 160cm2
Que 6. A vessel is in the form of a hemispherical bowl surmounted by a hollow cylinder of same diameter. The diameter of the hemispherical bowl is 14 cm and the total height of the vessel is 13 cm. Find the total (inner) surface area of the vessel. (Useπ= 22/7)
Ans 6.
Radius of hemisphere = 14/2 = 7 cm
Que 7. The largest possible sphere is carved out of a wooden solid cube of side 7cm. Find the volume of the wood left.
Ans 7. Side of cube a = 7 cm
The diameter of the largest possible
= side of the cube
Radius = 7/2 cm
Volume of the wood left = volume of cube - volume of sphere
Hence, volume of wood = 163.3 cm3
Que 8. A cone of height 20 cm and radius of base 5 cm is made up of modelling clay. A child reshapes it in the form of a sphere. Find the diameter of the sphere.
Ans 8. Radius of the cone = r = 5cm and
Height of the cone = h = 20cm
Let the radius of the sphere =R
As per given statement,
Volume of sphere = Volume of cone
4/3 πR3 = 1/3πr2h
4R3 = 5 x 5 x 20
R = 5 cm
Diameter of the sphere = 2R = 2 x 5 = 10 cm
Que 9. A metallic solid sphere of radius 10.5 cm is melted and recasted into smaller solid cones each of radius 3.5 cm and height 3 cm, How many cones will be made ?
Ans 9. Radius of given sphere = 10.5 cm
Hence, number of recasted cones = 126.
Que 10. What is the capacity of a cylindrical vessel with a hemispherical portion raised upward at the bottom?
Ans 10. Capacity of the given vessel
Chapter - Statistics
Que 1. Find the median class of the following distribution
| Class | 0−10 | 10−20 | 20−30 | 30−40 | 40−50 | 50−60 | 60−70 |
| Frequency | 4 | 4 | 8 | 10 | 12 | 8 | 4 |
Ans 1. First we find the cumulative frequency
| Classes | Frequency | Cumulative Frequency |
| 0−10 | 4 | 4 |
| 10−20 | 4 | 8 |
| 20−30 | 8 | 16 |
| 30−40 | 10 | 26 |
| 40−50 | 12 | 38 |
| 50−60 | 8 | 46 |
| 60−70 | 4 | 50 |
| Total | 50 |
Here, n/2 = 50/2 = 25
∴ Median class = 30 − 40.
Que 2. Find the sum of lower limit of median class and modal class of the following
| Distribution | 0-5 | 5-10 | 10-15 | 15-20 | 20-25 |
| Frequency | 10 | 15 | 12 | 20 | 9 |
Ans 2. Sum of lower limit of median class and modal class = 25 ←
Step-by-step explanation:
The given classes are 0-5, 5-10, 10-15, 15-20, 20-25
| Class Width | Frequency ( f ) | Cumulative Frequency |
| 0 - 5 | 10 | 10 |
| 5 - 10 | 15 | 25 |
| 10 - 15 | 12 | 37 |
| 15 - 20 | 20 | 57 |
| 20 - 25 | 9 | 66 |
So we have
∑f = 10 + 15 + 12 + 20 + 9 = 66
∑f = 66
Case 1:-
Median is the middle value.
⇒ The number of values are Even. So there are two middle values.
In this case the middle values are 33rd value and 34th value.
These values lie in the class 10 - 15
⇒ Lower limit of Median Class = 10
Case 2:-
Mode is defined as the value which occurs most frequently in a set of data. It indicates the most common result.
So frequency of the class 15 - 20 is the greatest.
So 15 - 20 is the Modal Class.
⇒ Lower limit of Modal Class = 15
Now according to the requirement
⇒ Sum of lower limit of median class and modal class = 10 + 15
→ Sum of lower limit of median class and modal class = 25 ←
Que 3. Daily wages of a factory workers are recorded as follows
Find the lower limit of the modal class
Ans 3. The given data is an inclusive series. So,
We convert into an exclusive form as follows
| Daily Wages | 130.5-136.5 | 136.5-142.5 | 142.5-148.5 | 148.5-154.5 | 154.5-160.5 |
| No of workers | 5 | 27 | 20 | 18 | 12 |
Clearly, the class 136.5 - 142.5 has maximum frequency,
so it is the modal class and its lower limit is 136.5
Hence 136.5 Ans.
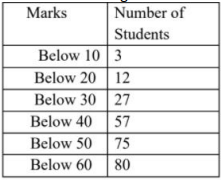
Que 4. For the following distribution find the modal class
Ans 4.
| Marks | Number of students | Class interval | Frequency |
| below 10 | 3 | 0−10 | 3 |
| below 20 | 12 | 10−20 | 12−3=9 |
| below 30 | 27 | 20−30 | 27−12=15 |
| below 40 | 57 | 30−40 | 57−27=30 |
| below 50 | 75 | 40−50 | 75−57=18 |
| below 60 | 80 | 50−60 | 80−75=5 |
Modal class is the class which has the highest frequency.
The highest frequency is 30 which is associated with the class 30−40
Therefore, the modal class is 30−40
Que 5. Find the mean of the following distribution
Ans 5.
Que 6. The arithmetic mean of the following frequency distribution is 25. Determine value of p.
| Class interval | 0−10 | 10−20 | 20−30 | 30−40 | 40−50 |
| Frequency | 5 | 18 | 15 | p | 6 |
Ans 6. Given Arithmetic mean =25
125=44+P
P=125−44
P=81
∴ value of P=81















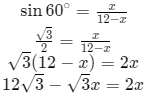





















Post a Comment